Limit

3. Limit#

3.1. Domain of functions#

a. Denominator cannot be 0 i. The domain of $f (x) = \frac{1}{\sqrt{x^{2} - 9}} \to x < - 3 \land x > 3$

b. Roots of even squared are greater than or equal to 0

c. The number of logarithm is greater than 0 ( $\ln x \to x > 0$ )

d. Arcsin $x$ and Arccos $x \to x \leq 1$

3.1.1. The equivalent of functions#

The same domain and the same mapping

i. $f (x) = \ln (x - 1) \neq g (x) = \ln \sqrt{(x - 1)^{2}}$

ii. $f (x) = \frac{x}{x} \neq g (x) = 1$

iii. $f (x) = \sqrt{x^{2}} = g (x) = | x |$

iv. $f (x) = {\begin{cases} - x, & x \geq 1 \\ 1, & x < 1 \end{cases} \neq g (x) = {\begin{cases} 0, & x = 1 \\ 1, & x < 1 \end{cases}$

3.1.2. The definition of limit#

Let $f (x)$ be a function defined on an interval that contains $x = a$ , except possibly at $x = a$ . Then we say that,

lim_{x \to a} f (x) = L

if for every number $ϵ > 0$ there is some number $δ > 0$ such that

| f (x) - L | < ϵ whenever 0 < | x - a | < δ

3.2. Solve the limit Problem#

3.2.1. Substitute Directly#

3.2.2. L’Hopital’s Law#

i. Infinity to infinity

ii. Infinity small to infinity small

lim_{x \to 1} \frac{x - 1}{x^{2} - 1} = lim_{x \to 1} \frac{1}{2 x} = \frac{1}{2}

3.2.3. The Property of infinity (∞) and infinity small (0)#

The product of infinity small with bounded function (e.g., $\sin x$ , $\cos x$ , $\arctan x$ , $a r c c o t x$ ) is infinity small.

lim_{x \to \infty} \frac{\sin x}{x} = lim_{x \to \infty} \sin x * \frac{1}{x} = 0

lim_{x \to 2} (x - 2) \sin \frac{1}{x - 2} = lim_{u \to \infty} \frac{\sin u}{u} = 0

3.2.4. Three special cases#

Factorization

lim_{x \to 1} \frac{x - 1}{x^{2} - 1} = lim_{x \to 1} \frac{1}{x + 1} = \frac{1}{2}

lim_{x \to 4} \frac{x - 4}{\sqrt{x + 5} - 3} = lim_{x \to 4} \frac{\sqrt{x + 5} + 3}{x + 5 - 9} = lim_{x \to 4} \sqrt{x + 5} + 3 = 6

Rationalization of numerator and denominator

lim_{x \to 4} \frac{x - 4}{\sqrt{x + 5} - 3} = lim_{x \to 4} \frac{(x - 4) (\sqrt{x + 5} + 3)}{(\sqrt{x + 5} - 3) (\sqrt{x + 5} + 3)}

Get the highest order $x \to \infty$

lim_{x \to \infty} \frac{2 x^{3} + 3 x^{2} + 5}{7 x^{3} + 4 x^{2} - 1} = \frac{2}{7}

lim_{x \to \infty} \frac{3 x^{2} + 5}{7 x^{3} + 4 x^{2} - 1} = 0

lim_{x \to \infty} \frac{2 x^{3} + 3 x^{2} + 5}{4 x^{2} - 1} = \infty

3.3. Two important limit#

i. $lim_{u (x) \to 0} \frac{\sin u (x)}{u (x)} = 1$

Prove using the definition of differential

a) $lim_{x \to 0} \sin x = x$

$f^{'} (x) = \frac{f (x + δ) - f (x)}{δ} \to f (x + δ) = f (x) + f^{'} (x) * δ, when δ \to 0$

$\sin (x + δ) \approx \sin x + \cos x * δ$ when $δ \to 0$

$\sin δ \approx 0 + δ$ , when $δ \to 0$

Replace $δ$ with $x$ , $lim_{x \to 0} \sin x = x$

ii. $lim_{A \to \infty} {(1 + \frac{1}{A})}^{A} = e$

Prove using Taylor’s Formula

$e < {(1 + \frac{1}{k})}^{k} = 1 + \frac{k}{1} {(\frac{1}{k})}^{1} + \frac{k (k - 1)}{1 \cdot 2} {(\frac{1}{k})}^{2} + \frac{k (k - 1) (k - 2)}{1 \cdot 2 \cdot 3} {(\frac{1}{k})}^{3} + \dots$

a)

$e = 1 + \frac{1}{2!} + \frac{1}{3!} + \dots = e$
$lim_{x \to \infty} {(1 - \frac{2}{x})}^{x} = e^{- 2} = \frac{1}{e^{2}}$
$lim_{x \to 0} (1 - 2 x)^{\frac{1}{x}} = e^{- 2}$
$lim_{x \to \infty} {(1 + \frac{1}{n})}^{n + 3} = e$

3.4. Equivalence of infinity small#

i. $lim_{x \to 0} \frac{\sin x}{x} = 1 \to \sin x \sim x (when x \to 0)$

ii. $1 - \cos x \sim \frac{1}{2} x^{2}$

Prove: $lim_{x \to 0} \frac{1 - \cos x}{\frac{1}{2} x^{2}} = \frac{\sin x}{x} = 1$

iii. $\ln (1 + x) \sim x$

Prove: $\ln (1 + x + δ) = \ln (1 + x) + \frac{δ}{1 + x} = \ln 1 + δ \to \ln (1 + δ) \approx δ when δ \to 0 and x = 0$
$lim_{x \to 0} \frac{\ln (1 + x)}{\sin 3 x} = \frac{2 x}{3 x} = \frac{2}{3}$

iv. $e^{x} - 1 \sim x$

v. $(1 + x)^{α} = 1 + α x$

Prove: $(1 + x + δ)^{α} = (1 + x)^{α} + (α δ) (1 + x)^{α - 1} \to (1 + δ)^{α} = 1 + α δ when δ \to 0 and x = 0$

3.5. Continuous#

3.5.1. Continuous in functions#

$f (x)$ has a definition at $x_{0}$
$lim_{x \to x_{0}} f (x) = f (x_{0})$

3.5.2. Example#

$f (x) = {\begin{cases} x^{2} + 2 a, & x \leq 0, \\ \frac{\sin x}{2 x}, & x > 0 \end{cases}$

is continuous at $x = 0$ , what’s the value of $a$ ?

Answer

$lim_{x \to 0} (x^{2} + 2 a) = 2 a$ , $lim_{x \to 0} \frac{\sin x}{2 x} = \frac{1}{2} = 2 a \to a = \frac{1}{4}$

3.6. The break points of functions#

The point with invalid definition
The point with no limits

a. $x = 3$ for $f (x) = \frac{x^{2} + 1}{x - 3}$ , with limit $= \infty$
The point with limit that does not equal to its function value

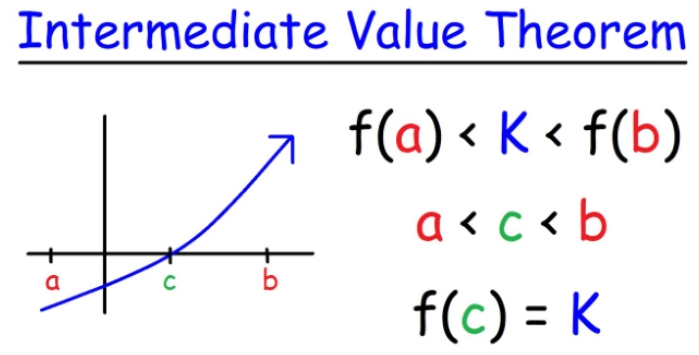
3.7. The Intermediate Value Theorem#

If $f (x)$ is continuous on the closed interval $[a, b]$ and $k$ is any number between $f (a)$ and $f (b)$ , then there exists at least one value $c$ in the interval $(a, b)$ such that $f (c) = k$ .

a. Prove there is a solution of $x^{3} - 4 x^{2} + 1 = 0$ on the interval $(0, 1)$ .