Matrix Interpretation

1. Matrix Interpretation#

1.1. Interpretation of equation sets#

Suppose we have the following equation sets

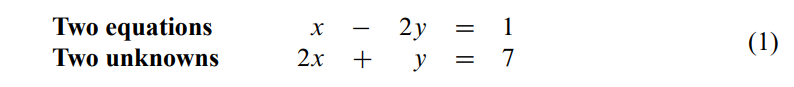
1.1.1. Row Picture#

Hard to draw when dimensions goes beyond 2

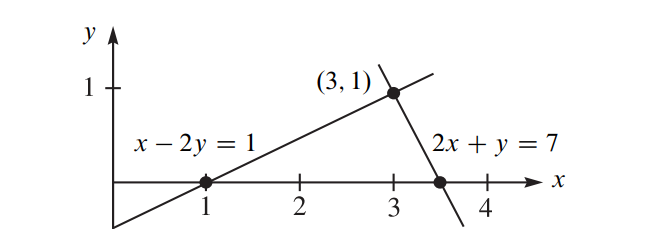
1.1.2. Column Picture#

Linear Combination of Columns

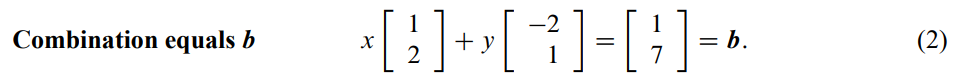
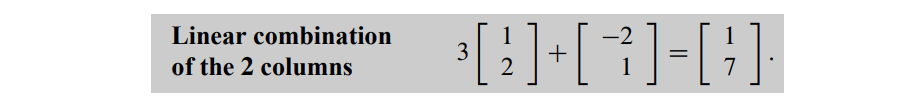
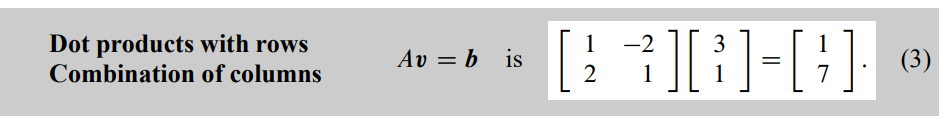
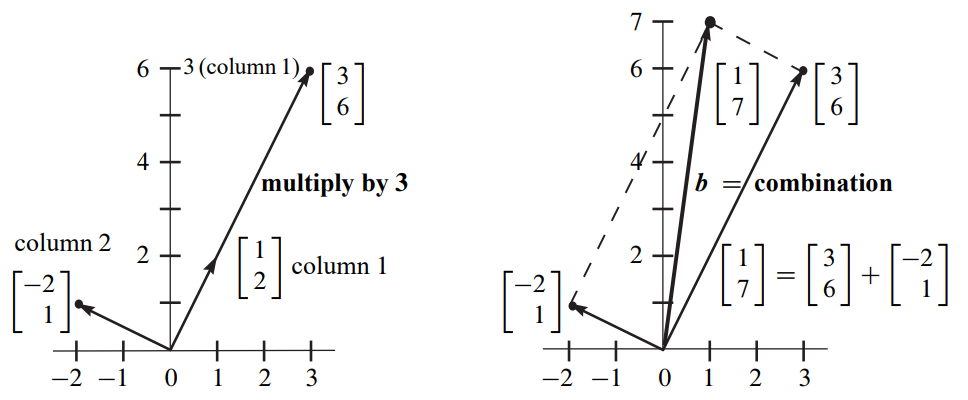
1.1.3. Quiz#

Q: Can I solve $A v = b$ for every $b$ ? (means, Do the linear combination of the columns fill 3D space?)

My Answer: Yes, if vectors in $A$ are not parallel, then they can be the basis for 3D space.

Professor’s Answer: If those three vectors lie within the same plane (which means you can get one vector from the linear combination of the other two vectors), then they can only form a plane instead of a 3D space.

1.2. Elimination#

A systematic way to solve the solution

1.2.1. Upper triangular matrix#

The most important purpose in elimination is from $A \to U$ (upper triangular matrix )

1.2.2. Augmented matrix#

Add y (response) into the x’s (parameters’) matrix

Method of Gaussian Elimination 3x3 Example

Given the system of equations:

\begin{array}{r} \begin{aligned} 4 x - 3 y + z & = - 8 \\ - 2 x + y - 3 z & = - 4 \\ x - y + 2 z & = 3 \end{aligned} \end{array}

We start with the augmented matrix:

\begin{array}{r} [\begin{array}{c} 4 & - 3 & 1 & | & - 8 \\ - 2 & 1 & - 3 & | & - 4 \\ 1 & - 1 & 2 & | & 3 \end{array}] \end{array}

Perform row operations to reduce to the row echelon form:

\begin{array}{r} [\begin{array}{c} 1 & - 1 & 2 & | & 3 \\ 0 & - 1 & 2 & | & 3 \\ 0 & 1 & - 7 & | & - 20 \end{array}] \end{array}

Further reducing to:

\begin{array}{r} [\begin{array}{c} 1 & 0 & 0 & | & - 2 \\ 0 & 1 & 0 & | & 1 \\ 0 & 0 & 1 & | & 3 \end{array}] \end{array}

This yields the solution:

\begin{array}{r} \begin{aligned} x & = 2 \\ y & = 1 \\ z & = 3 \end{aligned} \end{array}

1.2.3. Failure of Elimination#

Some pivot become 0, which means there are some vectors are parallel(dependent) to each other

1.2.4. Elimination matrix#

Use matrix to represent the elimination steps

\begin{array}{r} E_{32} (E_{21} A) = (E_{32} \cdot E_{21}) A = U (Associative law) \end{array}

$E_{21}$ means elimination row 1 using row 2

Again, matrix * vector = the combination of the columns of the matrix, therefore, matrix * column = column, matrix * row = row.

1.2.5. Permutation matrix $P$ #

Exchange the row

Exchange row 2 and row 1 $[\begin{matrix} 0 & 1 \\ 1 & 0 \end{matrix}]$ , example:

\begin{array}{r} [\begin{array}{c} 0 & 1 \\ 1 & 0 \end{array}] [\begin{array}{c} a & b \\ c & d \end{array}] = [\begin{array}{c} c & d \\ a & b \end{array}] (row operation) \end{array}

Exchange column 2 and column 1, example:

\begin{array}{r} [\begin{array}{c} a & b \\ c & d \end{array}] [\begin{array}{c} 0 & 1 \\ 1 & 0 \end{array}] = [\begin{array}{c} b & a \\ d & c \end{array}] (column operation) \end{array}

1.2.6. Inverse Matrix#

How we can reversing steps and transfer matrix from $U \to A$ ? (Inverse Matrix)

\begin{array}{r} [\begin{array}{c} 1 & 0 & 0 \\ 3 & 1 & 0 \\ 0 & 0 & 1 \end{array}] [\begin{array}{c} 1 & 0 & 0 \\ - 3 & 1 & 0 \\ 0 & 0 & 1 \end{array}] = [\begin{array}{c} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array}] \end{array}

$[\begin{matrix} 1 & 0 & 0 \\ - 3 & 1 & 0 \\ 0 & 0 & 1 \end{matrix}]$ means subtract $3 \times row1$ from row2. If we want to reverse the operation, we just need to add $3 \times row1$ to row2. That’s why we could easily calculate the inverse matrix.

1.2.7. Quiz#

Linear Algebra #2

Consider a linear equations

\begin{array}{r} {\begin{cases} 2 x + 2 y + 3 z = 3 \\ x - y = 2 \\ - x + 2 y + z = 1 \end{cases} \dots \dots \dots (1) \end{array}

Transform a matrix $A = (\begin{matrix} 2 & 2 & 3 \\ 1 & - 1 & 0 \\ - 1 & 2 & 1 \end{matrix})$ to an upper triangular matrix $(\begin{matrix} a_{11} & a_{12} & a_{13} \\ 0 & a_{22} & a_{23} \\ 0 & 0 & a_{33} \end{matrix}) .$

\begin{array}{r} [\begin{array}{c} 1 & 0 & 0 \\ - 0.5 & 1 & 0 \\ - 0.25 & 1.5 & 1 \end{array}] [\begin{array}{c} 2 & 2 & 3 \\ 1 & - 1 & 0 \\ - 1 & 2 & 1 \end{array}] = [\begin{array}{c} 2 & 2 & 3 \\ 0 & - 2 & - \frac{3}{2} \\ 0 & 0 & \frac{1}{4} \end{array}] \end{array}

1.3. Yalin Conclusion#

1.3.1. Row interpretation is suitable for first matrix, column interpretation is for second matrix [行前列后]#

$E_{r o w o p e r a t i o n} * A = U$
$A * E_{c o l u m n o p e r a t i o n} = U$

1.4. The meaning of Matrix pre-multiplication(矩阵左乘)#

$\begin{array}{r} [\begin{array}{c} 1 & 0 \\ 0 & 1 \end{array}] [\begin{array}{c} x_{1} \\ x_{2} \end{array}] = [\begin{array}{c} x_{1} \\ x_{2} \end{array}], \end{array}$

we can get that, in a plane with basis $(1, 0)$ , $(0, 1)$ , the vector $(x_{1}, x_{2})$ remains the same.
According to this, we can say

$\begin{array}{r} [\begin{array}{c} a_{11} & a_{12} \\ a_{21} & a_{22} \end{array}] [\begin{array}{c} x_{1} \\ x_{2} \end{array}] = [\begin{array}{c} b_{1} \\ b_{2} \end{array}] \end{array}$

indicates, after changing the plane basis from $(1, 0)$ , $(0, 1)$ to $(a_{11}, a_{21})$ , $(a_{12}, a_{22})$ , the coordinate of vector $(x_{1}, x_{2})$ becomes $(b_{1}, b_{2})$ .
Therefore, the matrix pre-multiplication is actually changing the basis.